How they measured their position

If we read the Derrotero of Francisco Albo, we can see that, whenever the weather conditions allowed him to, he measured the height of the sun at noon to determine the latitude. Let's explain how he did it taking this annotation as an example. It says as follows:

April 28, 1522. I measured the Sun at 36º, it had a declination of 17º, and it came to be scarcely 37 º height.

Latitude is one of the two coordinates needed to identify a point on the Earth's surface. It corresponds to the distance to the equator, being positive if it is to the North and negative if it is to the South, and it is measured in degrees. In the equator the latitude is zero, while in the North Pole 90º. Each degree is divided into 60 minutes, and these in turn in 60 seconds.

In the Derrotero of Francisco Albo we will see that it refers to the latitude as height of the pole, or simply height, which in this case should not be confused with the measured height of the sun above the horizon.

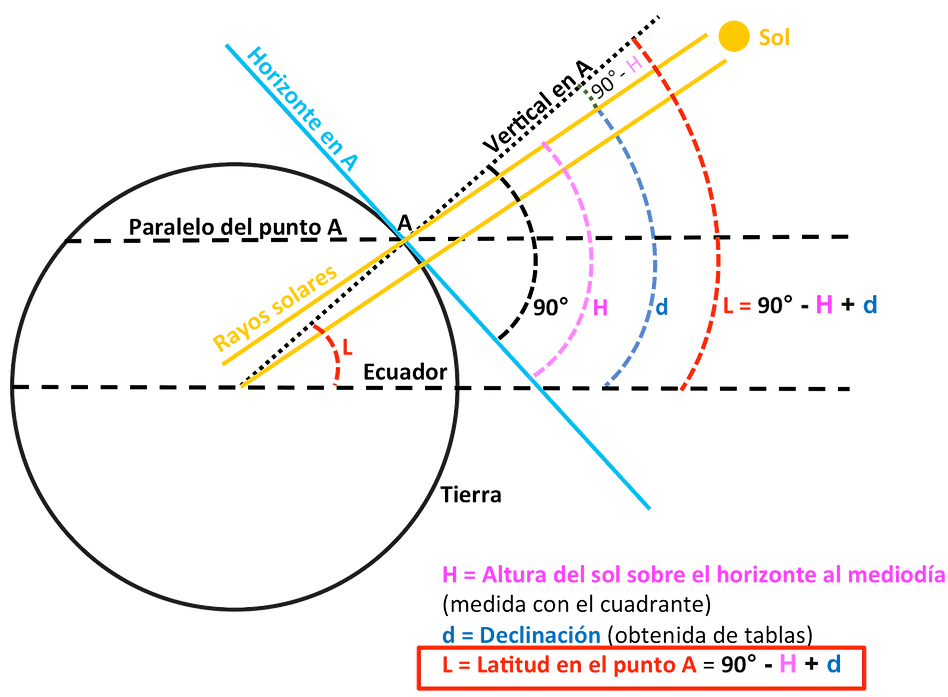
The measurement of the height of the sun at midday on the horizon was done through a very simple instrument called quadrant, innovative at this point of the 16th Century. It consists of a graduated 90-degree arc, from whose centre a weight hangs. If we point the side of the arc to the sun at 90 degrees, the reading on the line made by the weight will indicate the angle of its height above the horizon.

Classic naval quadrant used to measure the height of the Sun above the horizon.

Measuring the height of the sun on the horizon did not in itself provide any valid information on the position we occupy on the earth's surface, since every day of the year the sun reaches a different height at midday. To find the latitude using the sun as reference, we must consider that at any point on the Earth, the height reached by the sun at midday is variable throughout the year. This is due to the inclination of the Earth's rotation axis with respect to the plane that contains the Earth's orbit around the Sun, or of the ecliptic.

Therefore, if we want to find the latitude we must use another piece of information, called declination, which is the angle between the midday sun and the equator. We repeat: between the sun and the equator, not between the sun and the horizon as the sailors measured. The declination of the sun is different for each day of the year, and oscillates between a maximum angle of + 23º 45 'and a minimum of -23º 45' on the days of the summer and winter solstices respectively, being zero at the equinoxes.

Since the declination of the sun is independent of the place of observation and only depends on the date, to obtain the latitude we only have to measure the height of the sun on the horizon at noon, and add or subtract the declination corresponding to the date in which we are. In practice, the declination is a corrective dimension that we must apply to find the latitude depending on the date we are. We can understand it as how much more the sun rises in summer, and how much less the sun rises in winter.

From the Northern Hemisphere, the declination must be added between the spring and autumn equinoxes, and subtract it between autumn and spring according to the formula Latitude = 90º + Declination - Height above the horizon. In the Southern Hemisphere the sign will be reversed.

It is best understood with a sketch:

The pilots noted the daily declination of the Sun in some tables. Here, for example, you can download in pdf the Suma de Geographia, requested by the Emperor Charles V to Martín Fernández de Enciso, “Mayor of the mainland in the Western Indies” in 1518.

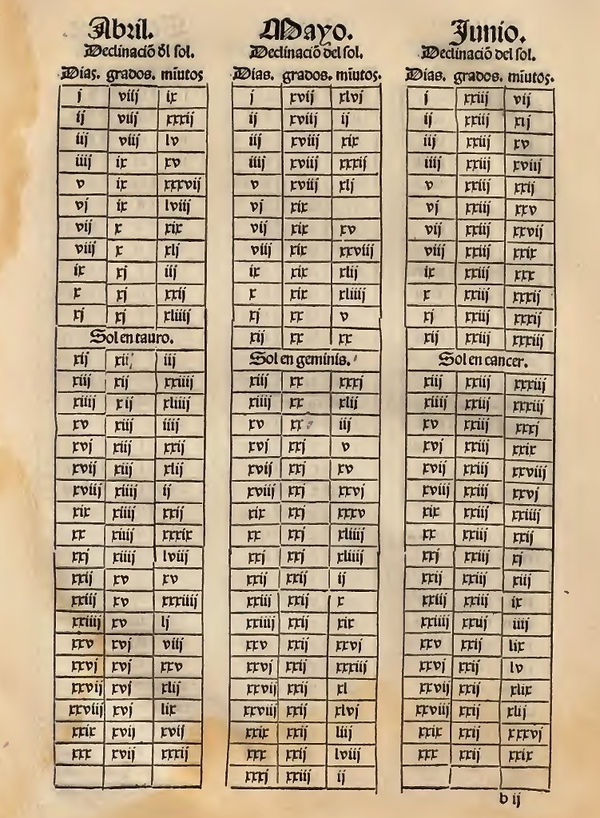
Table with the solar declinations, extracted from the Suma de Geographia, of Martín Fernández de Enciso, published in 1519 -although it is not the one he took during the expedition-. It is valid for the second year after the leap year, and therefore valid for 1522, used in the example.

We verified that, in the table, the corresponding declination to the day we are taking as an example, April 28, 1522, is 16º with 59 ', which practically coincides with that indicated by Francisco Albo in the Derrotero for this day, which he says to be 17th.

If we develop the complete formula to obtain the latitude with our example, we will see that it matches exactly the one determined by Francisco Albo:

Latitude = 90 + (-17º) - 36º = 37º of South latitude

The negative sign applied to the value of the declination corresponds to that of the date, April 28, but applied in the Southern Hemisphere, as in the Northern Hemisphere it would be positive. However, when navigating in the Southern Hemisphere it should be reversed and left as negative.

It is interesting to see how by then the equinoxes were March 11 and September 14. This is due to the fact that until the year 1582 the Julian calendar was in force, which was replaced by the Gregorian calendar that we continue to use today. In the former, a small error of 11 minutes was accumulated over the duration of each year. This made that after the 1257 years in which it was in force, a 10-day error accumulated. Therefore, in 1582 after Thursday, October 4, 1582 came Friday, October 15.

Through the slide, they could estimate the speed of the ship. Two men were needed. One of them would throw a rope over the stern, to the sea, with a log or a wooden board tied to it. The rope has knots in regular intervals. The other man has a hourglass. When the log is stable, the man holding the rope signals that he is going to let it go. The other starts the count with the hourglass. While the rope is being let go, the knots are counted until the time in the hourglass is over. Evidently, the more knots, the highest the speed of the ship. If we know the distance between the knots, we can also know the speed. This is the origin of the knot as a unit of speed in navigation.

The problem with this method of measuring speed is that the currents are not taken into account. If the ship moves in favour of the current, it will seem to advance less than it actually does. The sailors knew this perfectly well, but there was no way of resolving the issue.

However, the slide, as such, was not used until some years after this trip, but there were other similar systems to estimate the speed.

Slide and hourglass, used to measure speed at sea.

Determination of geographical length in navigation

Much is said that during the expeditions a method was known to find the geographical longitude, developed by Spaniards and Portuguese, which was kept under a scrupulous secret, hidden from the rest of the competing countries.

The truth is that we cannot establish the veracity of it. No document of the time describes this hypothetical method. On the contrary, the evidence that such a method did not exist is not only abundant, but even overwhelming. It was so secret that nobody knows anything about it even today. However, the idea of a supposed secret method is so appealing, that I fear we will have to continue denying it ad eternum.

The matter was resolved by estimation, or "with fantasy", by daily estimates of distances travelled at sea, whose accuracy depended fundamentally on experience and very rudimentary instrumentation, which among other things did not take into account the effect of currents. The sailing mastery of those navigators and pilots converted their estimates into sufficient, in such a way that sometimes their success is so incredible that they feed on the legend that "they must have known something more than what they tell". But were simply very good at what they did.

In the journey of the first circumnavigation we found a good example to deny that they knew a method to find the length. During the crossing of the Indian Ocean, Elcano is giving estimates of pending distances to the Cape of Good Hope. He suffers many days of storms and contrary currents, which difficult getting true estimates. So much so, that when he finally thinks he has passed the Cape - far from him, without seeing it - he goes north, but to his surprise he runs into the coast of South Africa beyond Port Elizabeth, more than 1,000 km east of where he thought he was. In this case, it was not just about making incorrect distance estimates. We must not forget that the length of the Moluccan Islands was in itself something very difficult to have determined exactly, both by Elcano and by the Portuguese who had already been there before.

Therefore, it is not only an accumulation of errors in the measurements of distances travelled daily throughout the Indian Ocean. Even the starting point, the Moluccas, must not have been located in its exact length.

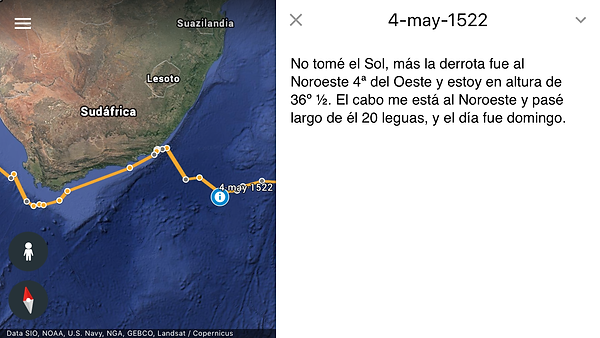
On May 4, 1522, Elcano thinks he has passed the Cape of Good Hope, but it is actually still quite far away. After veering north, he finds the South African coast.

We must add, however, that during the journey to Spain through the Atlantic, he often gives distance estimations to Tenerife that are very precise. Undoubtedly, he had good weather and favourable winds, and helped by the Benguela Current. He better located his position after passing the Cape of Good Hope, of which the length was known almost exactly by the numerous Portuguese expeditions, and therefore also by our expeditionaries.

Another good example is that of the return trip or Urdaneta, from the Philippines to Acapulco. There was great interest in being as exact as possible, and to that matter they had not only Urdaneta himself, but also the pilots Esteban Rodríguez and Rodrigo Espinosa. They all did their own measurements and estimations. At the end of the voyage, there was a total discrepancy among them of more than 1,000 km in the distance travelled to cross the Pacific.

Those who have read the journals of these trips will notice that the geographical longitude is never mentioned, which is another reason to deny the existence of the alleged secret method.

Another reason is that the King Philip III convened, at the beginning of the 17th Century, a competition for ideas on how to find the longitude. France, Holland and England would later do the same.

The issue of measuring the longitude on a ship was not “officially” solved until the 18th Century, when the English John Harrison invented a chronometer precise enough. As many times in science, the theoretical method was solved much earlier, but it had been impossible to apply it practically until the technological advance that the invention of the chronometer entailed. The hourglass, and the clocks that were invented after that- such as the pendulum-, were useful in land, but not in the sea. They depended on the gravity, and the movement of the ship was an issue. Harrison solved it through springs in the internal mechanism of the clock.

Measuring the difference in the geographical longitude between two points taking the stars as a reference implies measuring time. If we divide the 360 degrees of the terrestrial sphere by the 24 hours of the day, we obtain that each hour, the Earth turns 15 degrees. This is the premise for any calculation.

For example: I am in Seville, and exactly when I see that the Sun reaches its highest point in the sky I start a chronometer. The following day, when the Sun reached again its highest point, I will be able to see that 24 hours went by. However, if I move in the East-West direction, to Rome, for example, and I take with me my chronometer while it is still running, I will observe that the Sun reaches its highest point an hour and several minutes before than in Seville. Thus, we can rightly say that the longitude of Rome, with regard to Seville, is of a bit more than 17º.

Hernando Colón, son of Admiral Cristóbal Colón, already proposed in 1524 to equip the ships with watches to compare the times following this method. However, since there was no way for the clocks to measure well, the method failed. Therefore, efforts were made to find other methods to facilitate the matter:

Alonso de Santa Cruz, in his Book of Longitudes, of 1555, describes the method of the eclipses of the sun and the moon; but because they were very infrequent, the calculations were difficult, and their beginning and end were hard to establish, it was only useful to him in the islands and continents in order to locate them well in the letters. "... That the eclipses were well calculated by men trained in astrology before their departures to know precisely the day and hour and point of it, in which they had to start or end such eclipses, they could find out quite precisely the length of any place based on the point where they left."

King Philip II ordered in the year 1577 for measurements to be taken from all parts of the Empire, profiting that two eclipses of the moon were planned for that year. It was also useful to determine the size of the territories.

Galileo will discover the four main satellites of Jupiter in 1610. Then its transits and occultation on the disk of the planet would be discovered, and its observation is soon used in a similar way to the eclipse method of sun and moon, comparing the predicted moment for the phenomenon with the actual moment of its observation depending on the places from where it has been observed, with the great advantage that on Jupiter these phenomena occur daily. However, these methods were not feasible on the deck of a ship, but were instead used on land. From a ship it is absolutely impossible to keep Jupiter still in the lens of a 20-magnification telescope.

This method was followed by another that was based on the measurement of angular distances between the moon and different stars. It required complex calculations, which were simplified with the use of astronomical tables. Hence, the royal observatories of the different armadas were developed.

Therefore, we conclude that this scientific effort to determine the geographical longitude as accurately as possible was an obstacle race. It was first resolved with success in the mainland, but it would only be implemented on ships after the invention of the chronometer.

How they measured their position

Slide and hourglass, used to measure speed at sea.

​